

We only know the physics of our 3D-world (4D-spacetime if you want, but there are no four spacial dimensions, but one is temporal) Of course we cannot talk physically about a 4D-light source and light traveling through 4D-space and hitting a 3D-wall and casting a 3D-shadow. Light is cast on a 3D-object and blocked out by it and you try to reconstruct its shape from the light and dark spots left by it on the wall behind it. But a projection is essentially the same as a shadow image on the wall. The inner cube is becoming the outer one, becoming bigger because it is becoming the "front cube". You see the same for the rotating tesseract. And during this process it even loses its shape as a square. During the process of rotation, the back face is becoming the front face, hence shifting its size. But again, take the anagoly of a rotating 3D-cube in a picture. It seems like the complete structure is shifting, nothing is rigid at all. A shape might not be kept.Įven more interesting: look at this picture of a rotating tesseract. These cubes are distorted, and cannot be recognized as cubes. It might be hard to see, but there are "cubes" connecting the inner and outer cube.

The "outer" cube is the front cube for the analogue reason. It is the farthest away from you, hence the smallest. The small cube "inside" is the "back-cube" of the tesseract. They look as much as a cube as a square looks like a square in the 2D-depiction of a 3D-cube. But good luck finding these cubes in this picture. Actually a tesseract (technical term for 4D-cube) is composed of eight identical cubes. For example, you see a small cube inside, and a large cube outside. You recognize that it is composed of several cubes. Look at your favorite "picture" of a 4D-cube. We use the same analogies for projecting 4D-objects into 3D space (and then further into 2D-space to make an image file out of it). Also it is no longer in the form of a square, but you still recognize it as such.
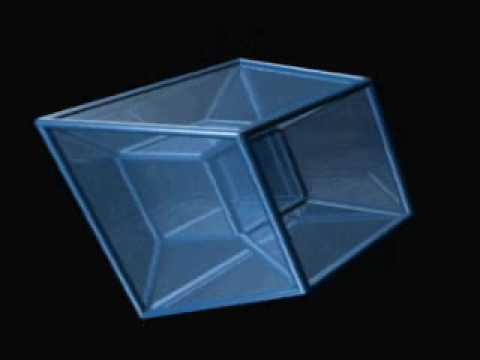
However, any (perspectively correct) image shows some face (the back face, if visible) as smaller as the front face. You know that a cube is composed of faces of the same size. For example: you know things are smaller if they are farther away.
Well, you (or your brain) have different techniques to reconstruct the 3D-nature of the object from this simplified representation. How is your brain achieving this? There is obviously information lost during the projection into 2D. Also you intuitively understand its shape and positioning in space. If you look at an image of a cube, the image is absolutely 2D, but you intuitively understand that the depicted object is some 3D-model. I will avoid using formulas as long as possible. I will keep things simple and will talk more about the philosophy and visualization than about the math behind it. The latter one might help us understand the generalized properties of such objects, but does not help visualizing it for us 3D-beings in a familiar way. All we can do is using tricks an analogies, and of course, the vast power of abstract mathematics. First things first: your brain is simply not made to visualize anything higher than three spacial dimensions geometrically.


 0 kommentar(er)
0 kommentar(er)
